

Step 2: SimplifyThen, the derivative of x2 is 2x: The derivative of ln x is 1/x, so the derivative of ln x2 is 1/x2 times the derivative of x2: Step 1: Differentiate with the Chain Rule Thus, using the chain rule, the derivative of sin x2 is cos x2 times 2x or just 2x cos x2. And then, we multiply by the derivative of the argument. In this example, the derivative of sin x2 is cos x2. To use the chain rule, we imagine the function has a simple argument and we write the derivative. If the argument were simply x, we’d differentiate sin x and get cos x. The function is sine and the argument is x2. Let’s say we have a function with a complicated argument, like sin x2. How does the chain rule work? Well, first of all, the chain rule is a formula for figuring out the composition of two or more functions. Let’s look at our first method, the chain rule. ln ( x – y ) ln(x–y) DOES NOT EQUAL ln ( x ) – ln ( y ) ln(x)–ln(y) for a function with subtraction inside the natural log, you need the chain rule.ĭerivative Of lnx^2 The Steps to Calculate.ln ( x + y ) ln(x+y) DOES NOT EQUAL ln ( x ) + ln ( y ) ln(x)+ln(y) for a function with addition inside the natural log, you need the chain rule.Values like ln ( 5 ) ln(5) and ln ( 2 ) ln(2) are constants their derivatives are zero.In certain situations, you can apply the laws of logarithms to the function first and then take the derivative.The derivative of ln ( x ) ln(x) is 1 x 1x.Remember the following points when finding the derivative of ln(x): So what’s our solution? The derivative of ln(x) is 1 / x. Ta-da! Now, we see that d/dx ln(x) = 1/x, and now we know why this formula for the derivative of ln(x) is true. We’re going to use this fact to plug x into our equation for e y.
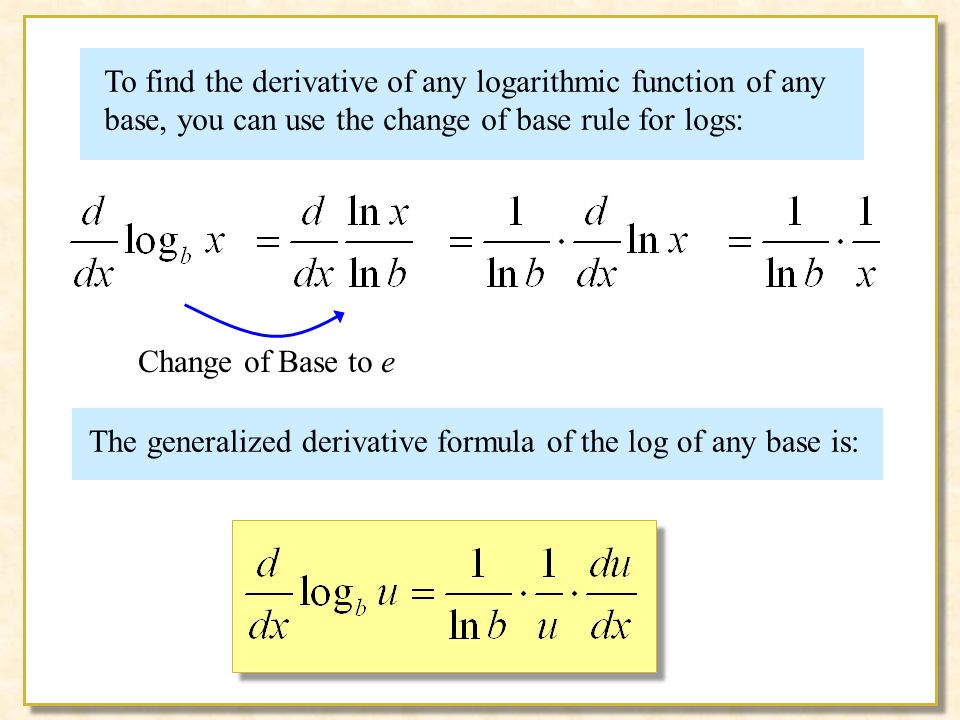
The last thing is to recall that y = ln(x) and plug this into our equation for y.We have (e^ y) dy/dx = 1. We’re getting super close now! Are you as excited as I am? We can divide both sides of this equation by x to get dy/dx = 1/x. On the right-hand side we have the derivative of x, which is 1. Therefore, by the chain rule, the derivative of e y is e^y dy/dx. Since the derivative of e to a variable (such as e ^x) is the same as the original, the derivative of f'(g(x)) is e ^y. The left hand side of the equation is e ^y, where y is a function of x, so if we let f(x) = e ^x and g(x) = y, then f(g(x)) = e ^y. The chain rule is a rule we use to take the derivative of a composition of functions, and it has two forms. We use the chain rule on the left-hand side of the equation to find the derivative. Okay, just a few more steps, and we’ll have our formula! The next thing we want to do is treat y as a function of x, and take the derivative of each side of the equation with respect to x. Therefore, by the definition of logarithms and the fact that ln(x) is a logarithm with base e, we have that y = ln(x) is equivalent to e^y = x. The definition of logarithms states that y = log b (x) is equivalent to b y = x.
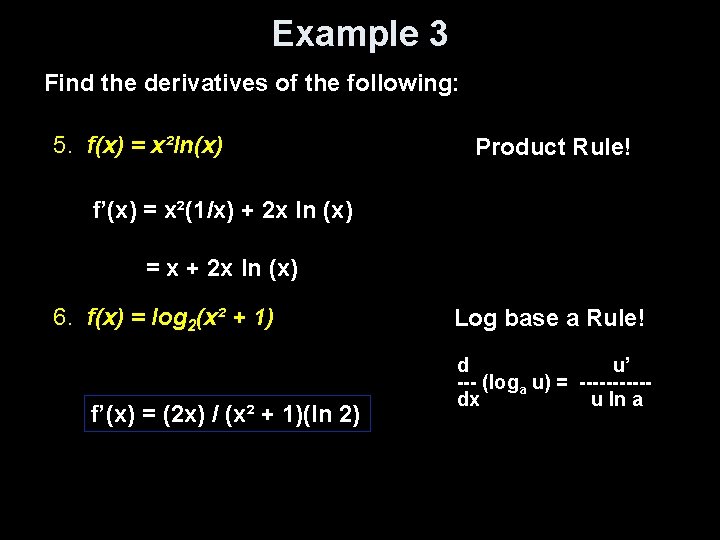
Next, we use the definition of a logarithm to write y = ln(x) in logarithmic form. To find the derivative of ln(x), the first thing we do is let y = ln(x). However, it’s always useful to know where this formula comes from, so let’s take a look at the steps to actually find this derivative. The derivative of ln(x) is 1/x and is actually a well-known derivative that most put to memory.


 0 kommentar(er)
0 kommentar(er)
